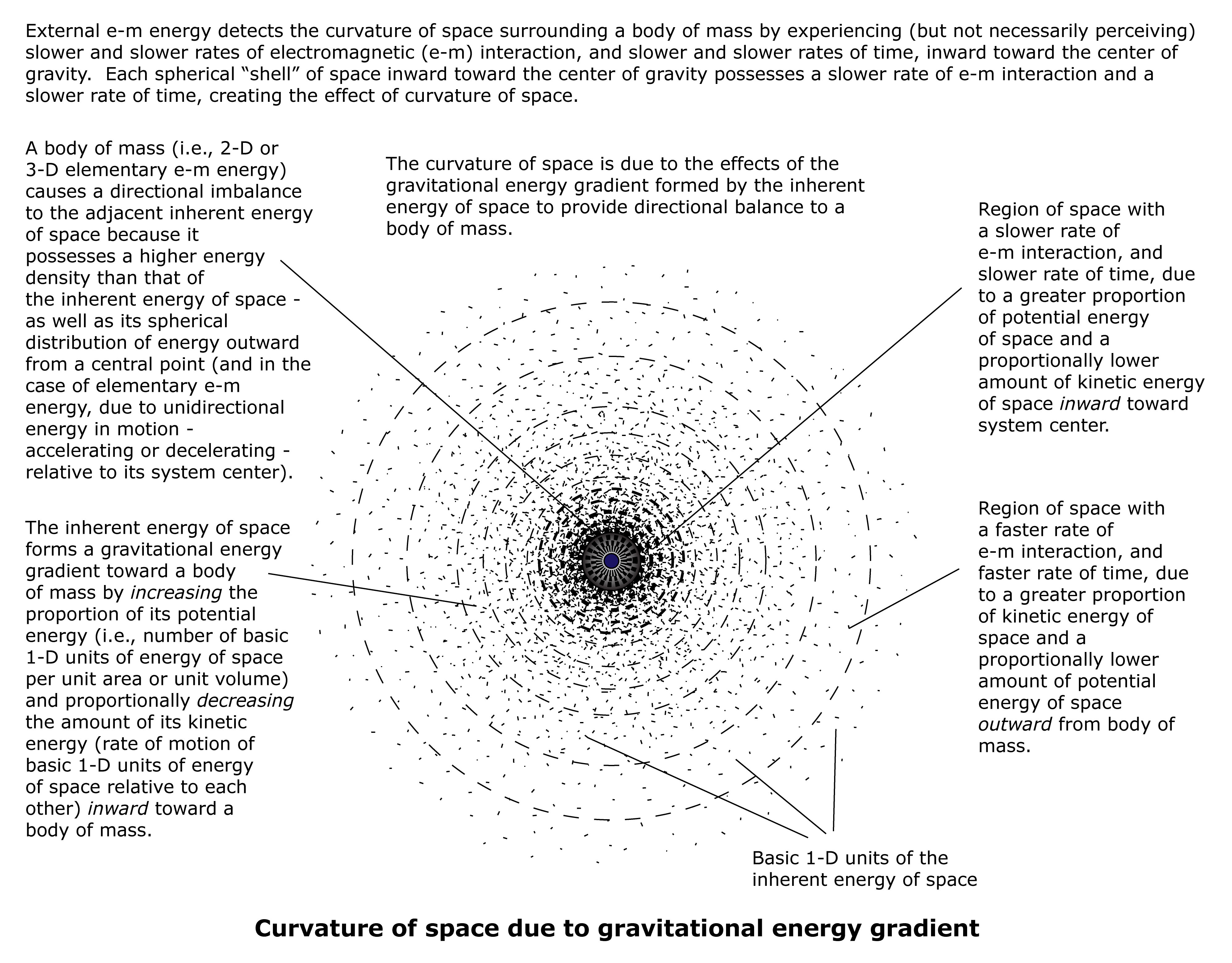
In this model, the curvature of space is due to the varying ratio of the amount of potential energy of space to the amount of kinetic energy of space approaching a body of mass.
A body of mass (including 2-D and 3-D elementary particles) creates an energy imbalance relative to adjacent space due to a differential in energy density, or due to motion (acceleration or deceleration) of energy relative to its system center. To provide directional balance to a body of mass, the inherent energy of space increases its potential energy (the number of basic 1-D units of space per unit area or unit volume) and proportionally decreases its kinetic energy (the rate of motion of basic 1-D units of space relative to each other) nearer and nearer to the center of gravity of a body of mass.
As the potential energy of space increases toward a body of mass, the rate of e-m interaction, or rate of time, slows down. The result is a gravitational energy gradient consisting of a higher rate of e-m interaction or faster rate of time further from the body of mass, with a decreasing rate of e-m interaction or slower rate of time closer and closer to the body of mass.
The properties of the energy of space essentially create curved space surrounding a body of mass. Each spherical radius level (”shell”) of space surrounding a body of mass possesses a different rate of e-m interaction, or rate of time. External e-m energy will detect this curvature by experiencing the differential in rate of e-m interaction, or rate of time, within a gravitational energy gradient.
(Since time is produced in electromagnetic interactions, when a gravitational energy gradient is so strong that rate of time = 0 (as it is at the event horizon of a black hole), this means that electromagnetic interactions are not occurring. If e-m energy is not active, what form of unidirectional energy exists in such a region to provide directional balance? Only directionally opposing gravitational energy gradients interacting with each other?)
