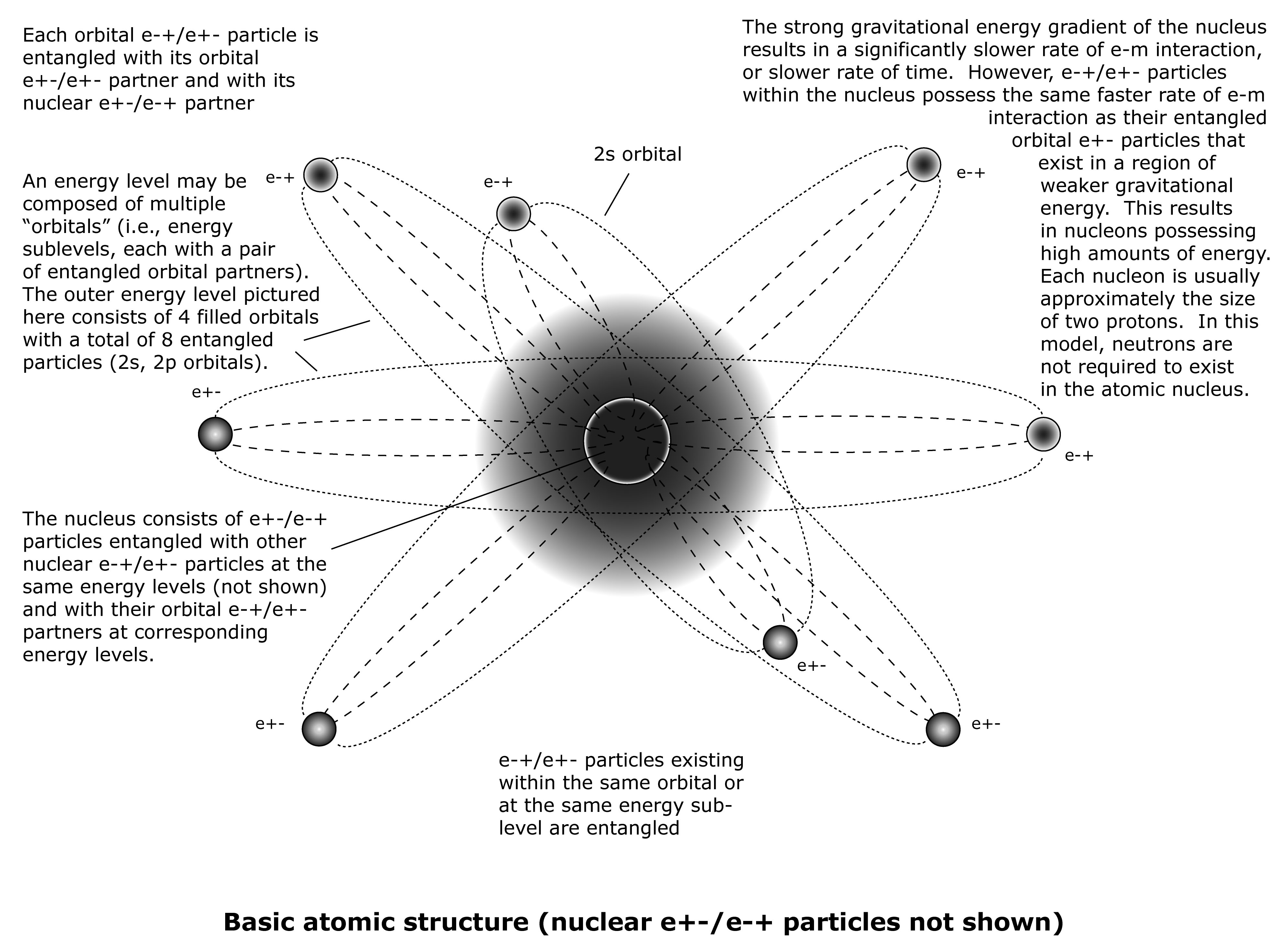
An atomic energy system is composed of a nucleus consisting of e-+/e+- particles entangled with nuclear e+-/e-+ particles and with orbital e+-/e-+ particles at corresponding energy levels at relatively great distances from the nucleus (Note: e-+/e+- designates that the elementary particle is alternating between [-] and [+] e-m directionality with every e-m interaction).
An atomic nucleus has a very small diameter analogous to the Schawarzchild radius of large bodies of mass due in part to 2-D and 3-D unidirectional energy in motion relative to system center. The energy within an atomic nucleus is directionally balanced by magnetic energy, time energy, and a gravitational energy gradient. The gravitational energy gradient is very strong inside the atomic nucleus and inside its constituent e-+/e+- nucleons.
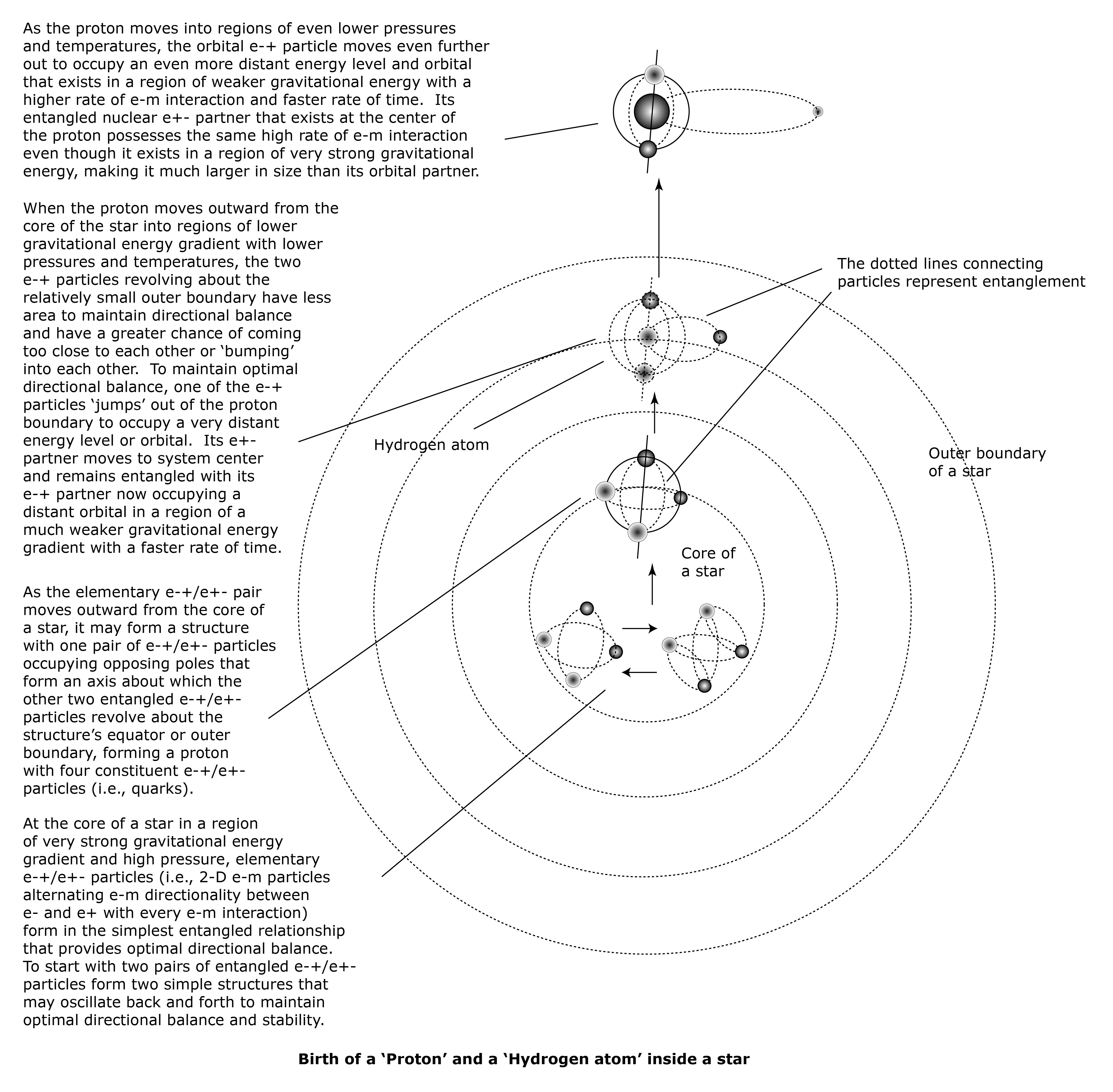
Let’s start with the most basic atomic structure – a Hydrogen atom with a ‘proton’ nucleus. The constituent e-+/e+- particles (i.e., quarks) within a proton are entangled to provide each other directional balance. These entangled relationships begin with the very ‘birth’ of atomic energy systems within the core of a star, changing structure as they migrate outward toward lower and lower pressures and temperatures. At the core of a star, the proton is formed, but with 4 constituent e-+/e+- particles (i.e., quarks), somewhat resembling a neutron. Two of the constituent e-+/e+- particles are entangled, each existing at one of the poles of the proton. The other two are entangled with each other, revolving about the equator of the proton. As the proton moves away from the core of a star into regions of lower and lower pressure and temperature, the two entangled e-+/e+- particles revolving about the relatively small outer boundary of the proton have a higher probability of ‘bumping’ into each other, not existing in a large enough region to provide optimal directionality to each other.
To attain a more stable directional balance, one of these nuclear e+-/e-+ particles (i.e., quark) moves to occupy the center of the proton in the region of strongest gravitational energy gradient while its entangled nuclear e-+/e+- partner ‘jumps’ outside the proton boundary to occupy a very distant energy level or orbital. This orbital e-+ particle now exists in a region of much weaker gravitational energy gradient with a faster rate of e-m interaction, and correspondingly faster rate of time. The orbital e-+ particle remains entangled with its nuclear e+- particle (i.e., quark) now existing at the center of the proton in a region of very strong gravitational energy gradient. The nuclear e+- particle takes on the same high rate of e-m interaction as its entangled orbital e-+ partner. The nuclear e+- particle existing in a region of strong gravitational gradient with a slower rate of e-m interaction and correspondingly slow rate of time, but with the same high rate of e-m interaction as its orbital e-+ partner, will be much larger in size than its distant entangled orbital e-+ partner.
The Hydrogen atom is the building block of all other atoms. It only possesses one proton in its nucleus. In this model, it is possible, and probable, that atomic nuclei are composed of nucleons of different sizes due to a combination of entangled relationships and gravitational gradient strength. The size of nucleons probably have mass-energy equal to 1 X proton mass (as in the Hydrogen nucleus), 2 X proton mass (the most common size nucleon), and 3 X proton mass. While neutrons are not required in atomic nuclei, they can be ‘formed’ and ’emitted’ from atomic nuclei under high energy conditions, such as those in large nuclei. The structure of atomic nuclei (without neutrons) is discussed in more detail at entanglednucleons.net.
In all atoms, the nucleus is surrounded by distant orbital e-+/e+- particles that occupy various energy levels and orbitals. Each orbital e-+ particle is entangled with an e+- nucleon that exists at a corresponding energy level within the nucleus. If the orbital e-+ particle occupies an orbital with another e+- particle, then the two will also be entangled. The ‘ideal’ atom has complete directional balance. That is, its nucleons and orbital particles are all entangled, providing optimal directional balance to the atomic structure. Of course, these atoms will be less likely to interact with other atoms to achieve greater directional balance since they already have optimal directional balance (e.g., inert gases).