The inherent energy of space can provide directional balance to ‘intruding’ nonrandom or unidirectional energy through one or more of the following methods:
-
- formation of magnetic energy
- formation of time energy
- formation of a gravitational energy gradient
Here, the gravitational energy gradients formed by the inherent energy of 2-D space to provide directional balance to an unentangled 2-D electron and to an unentangled 2-D positron are compared relative to the e-m directionality of each (see illustrations).
Unidirectional energy in motion relative to its origin or system center will result in the formation of magnetic energy and time energy by the inherent energy of space to provide directional balance. Elementary 2-D electric energy, for example, consists of unidirectional energy confined to a 2-D circular plane that moves either inward toward system center or outward away from system center. This motion results in the formation of 2-D magnetic and 2-D time energies to provide directional balance.
But there is also a difference between the energy density of 2-D electric energy and that of the inherent 2-D energy of space. Energy density isn’t something that necessarily moves or changes relative to system center (e.g., in large bodies of mass), but it does create a unidirectional energy gradient or differential with that of the surrounding inherent energy of space. Large bodies of mass that are electrically neutral will be directionally balanced by relatively static gravitational energy gradients (i.e., not in motion relative to system center).
But here, unentangled elementary 2-D e-m energy systems, such as an electron and a positron, will be considered. In these cases, the 2-D electric energy does move through a gravitational energy gradient relative to system center.
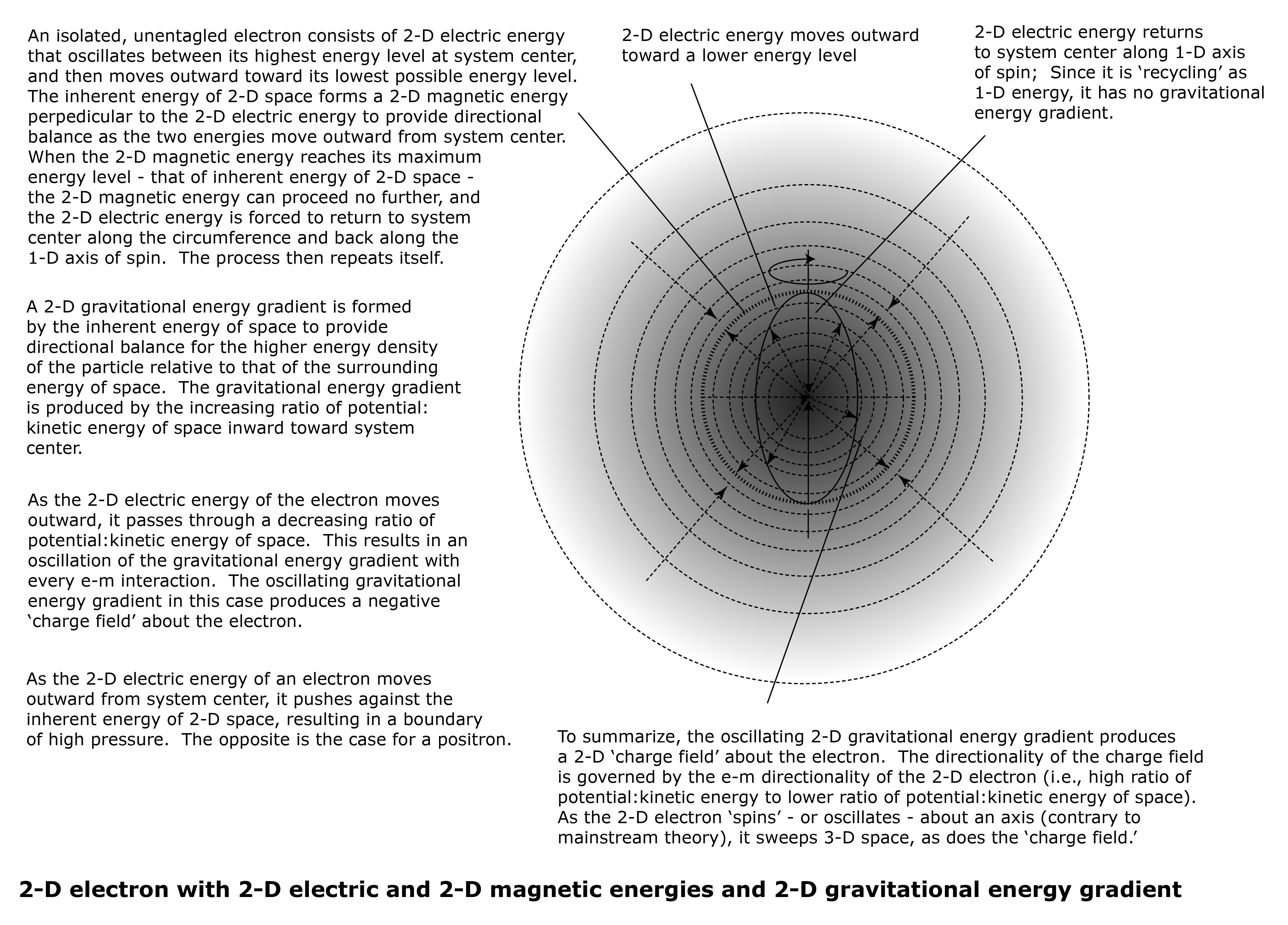
In the case of an unentangled, or isolated, electron, its 2-D electric energy moves outward from system center toward a lower energy level. As it does, it pushes against the inherent 2-D energy of space, resulting in higher pressure at the outer boundary. The energy density of the 2-D electron relative to that of adjacent 2-D energy of space is directionally balanced by the formation of a 2-D gravitational energy gradient through an increasing ratio of potential:kinetic energy of space inward toward system center. So as the 2-D electric energy of an electron moves outward through the gravitational gradient, it moves from a higher ratio of potential:kinetic energy of space toward a lower and lower ratio of potential:kinetic energy. This results in an oscillation or vibration of the gravitational energy gradient with every e-m interaction of the 2-D electron, and produces a negative ‘charge field’ about the electron.
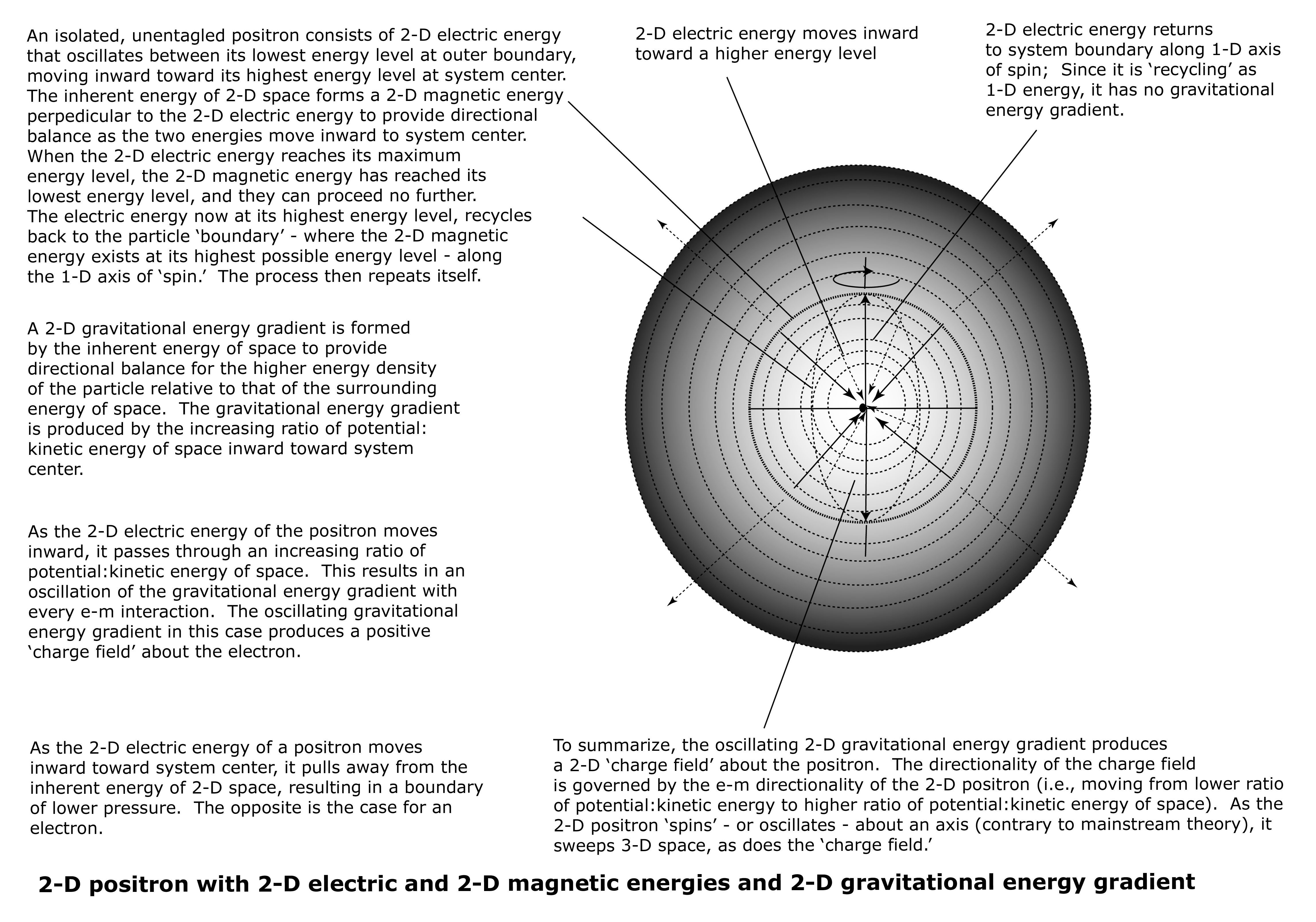
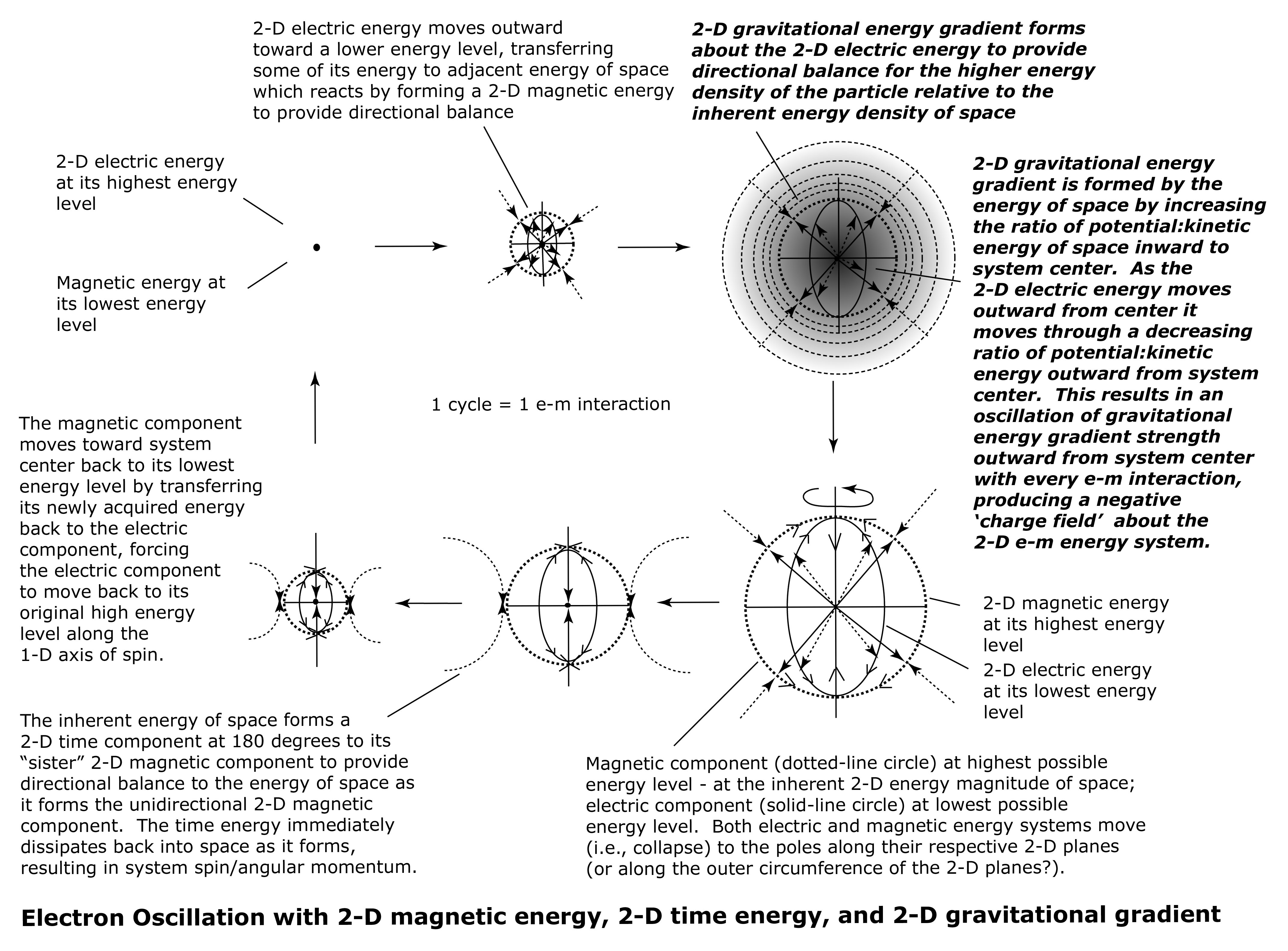
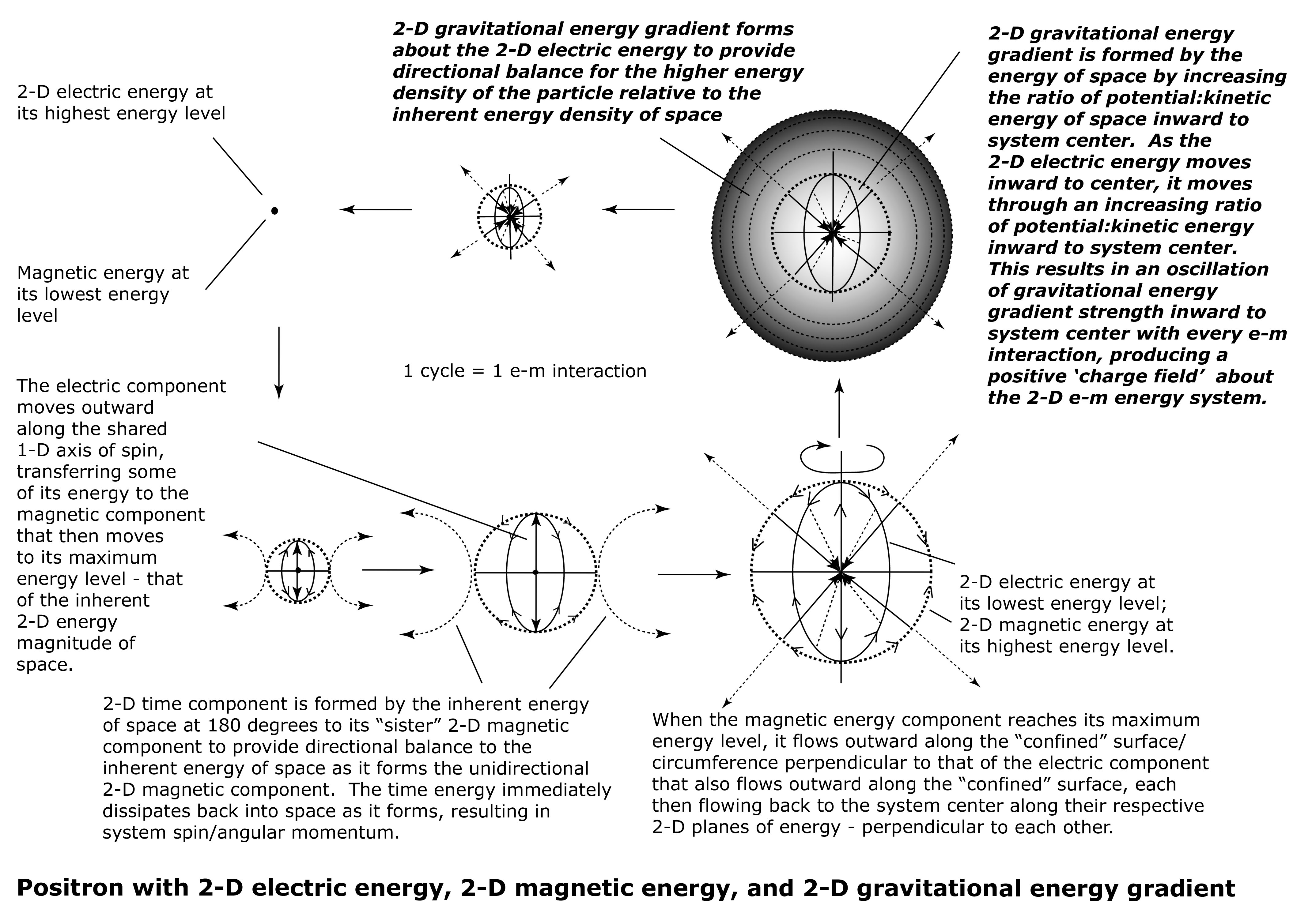
In the case of an unentangled, or isolated positron, its 2-D electric energy moves inward to system center toward a higher energy level. As it does, it pulls away from the inherent 2-D energy of space, resulting in lower pressure at the outer boundary. The energy density of a 2-D positron relative to that of adjacent 2-D energy of space is directionally balanced by a 2-D gravitational energy gradient formed through an increasing ratio of potential:kinetic energy of space inward toward system center. So as the 2-D electric energy of a positron moves inward through the gravitational energy gradient, it moves from a low ratio of potential:kinetic energy of space toward a higher and higher ratio of potential:kinetic energy of space. This results in an oscillation of the gravitational energy gradient with every e-m interaction of the 2-D positron, and produces a positive ‘charge field’ about the positron.
So the inherent 2-D energy of space reacts to provide directional balance in both cases, but in different directions relative to system center. The total energy of a gravitational energy gradient is equal to the total energy of the body of mass for which it provides directional balance. So the range of a gravitational energy gradient is limited.
Unlike entangled 2-D particles, unentangled 2-D particles go through two phases during each electromagnetic (e-m) interaction: 1) 2-D electric energy moves toward or away from system center, and 2) the electric energy recycles in the form of 1-D electric energy away from or toward system center. It is the 2-D phase that results in the formation of a gravitational or inverted gravitational energy gradient to provide directional balance. The 1-D phase does not possess a gravitational energy gradient since such a gradient can only form in 2-D or 3-D space by varying its ratio of potential:kinetic energy of space relative to 2-D or 3-D system center.
See the structures for unentangled electron and unentangled positron below, along with their 2-D magnetic energies and 2-D gravitational gradients that form to provide directional balance to the motion and energy density of the 2-D electric energy.
An unentangled 2-D electron has the following structure.
An illustration of the unentangled electron structure is enlarged below:
An unentangled positron structure is illustrated below.
An illustration of the unentangled structure of a positron is enlarged below.